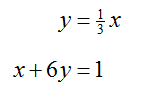Solving a System of Two Linear Equations Algebraically
In Section 2.1, we solved several systems of linear equations by graphing each linear equation in the system. The solution to the system is any ordered pair that satisfies all of the equations. On the graph, this ordered pair is the point where all of the equations intersect.
Using a graph to find a point of intersection has limitations. If the graph is on a piece of paper, it is difficult to accurately locate the point of intersection using the scales on the axes of the graph. If you constructed the graph, you have to be extremely careful and precise to get a valid approximate answer.
A graphing calculator can draw a more accurate graph (as long as you enter the equations correctly), but the algorithms in the calculator are approximate. This means that the location of the point of intersection may be exact or it may be very close to the exact point of intersection.
Suppose we want to solve the system of linear equations
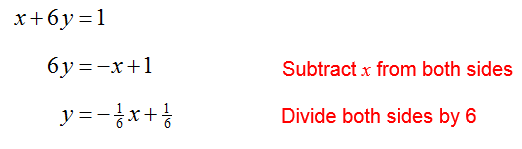
To solve this system graphically, we need to solve each linear equation for y. The first equation is already solved for y. To solve the second equation for y,
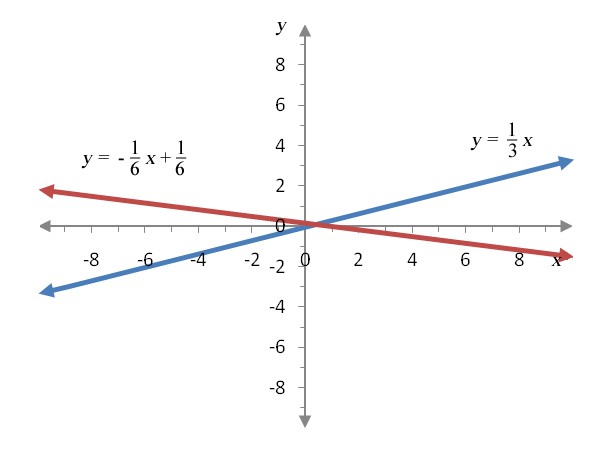
Let’s look at a graph the system to find the point of intersection.
Figure 1 – A graph of a system whose point of intersection is about (0.5, 0).
From the graph, the solution to the system looks to be approximately (0.5, 0). We can check this solution by substituting x = 0.5 and y = 0 and into the original system of equations:
Since neither equation is true, the estimate of the point of intersection is not exact. To find an exact solution, we’ll need to use an algebraic strategy to solve the system of equations.
Read in Section 2.2
Section 2.2 Workbook (PDF) – 9/2/19
Watch Video Playlist