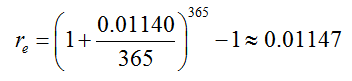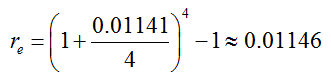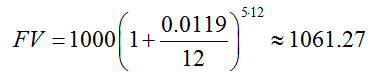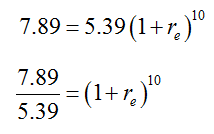What is an effective interest rate?
The amount of interest compounded depends on several factors. The nominal rate r and the number of conversion periods m both influence the future value over a predetermined time period. A savings account earning a higher nominal rate over fewer conversion periods might have the same future value as another savings account with a lower nominal rate and a higher number of conversion periods. To help us compare nominal interest rates, we use the effective interest rate. The effective interest rate is the annual interest rate that leads to the same future value in one year as the nominal interest rate compounded m times per year.
The effective interest rate is
where r is the nominal interest rate, and m is the number of conversion periods per year.
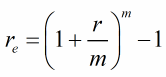
Another name for the effective interest rate is the effective annual rate or annual percent yield (APY).
Example 5 Best Investment
An investor has the opportunity to invest in one of two opportunities. The first opportunity is a certificate of deposit (CD) earning 1.140% compounded daily. The second opportunity is an investment yielding a dividend of 1.141% compounded quarterly. Which investment is best?
Solution The better investment is the one with the higher effective interest rate. The nominal rate for the CD is r = 0.01140. Interest is earned on a daily basis so m = 365. This gives an effective rate of
For the other investment, r = 0.01141 and m = 4. The effective rate for this investment is
The effective rate for the CD, 1.1147%, is higher than the effective rate for the investment, 1.1146%. Because of this, the CD is the better investment.
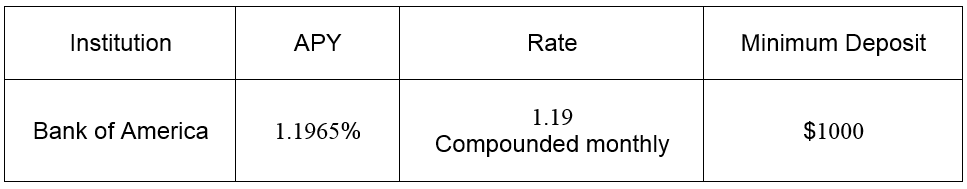
By law, the effective rate of interest is shown in all transactions involving interest charges. The APY is always prevalent in advertisements, such as the one below for five-year CD rates from Bankrate.com on December 29, 2011.
We can also use the APY to compute accumulated amounts. Suppose we want to compute the future value from depositing $1000 in the Bank of America five year CD. We could calculate the future value using the rate,
Alternatively, we compute the future value using the APY and compound annually,
This gives us another way of computing accumulated amounts.
The future value FV compounded at an effective interest rate (APY) of re is
where PV is the present value or principal, and t is the term in years.
Since the APY is always shown in financial transactions, this formula allows us to compute accumulated amounts from the APY.
We can also use the compound interest formula to find the rate at which an amount grows. In this case, we think of PV as the original amount and FV as the amount it grows to.
Example 6 Growth of Ticket Prices
In 2000, the average price of a movies theater ticket was $5.39. In 2010, the average price increased to $7.89. At what effective percentage rate did prices increase over the period from 2000 to 2010 on average?
Source: National Association of Theater Owners
Solution The original price in 2000 is $ 5.39. This price grows in ten years to $7.89. Use these values in to find the effective rate :
To solve for , remove the tenth power by raising both sides of the equation to the one-tenth power.
Over this period, the price of tickets increased by an average of 3.88% per year.