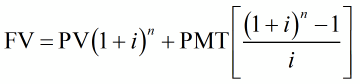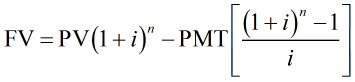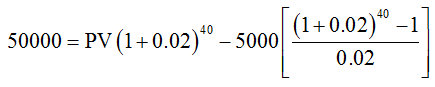How do you find the present value of an annuity?
In Section 5.3, we were able to calculate the future value or payments of annuities that were increasing in value or decreasing in value. For an ordinary annuity whose present value is PV, the future value is
if the payments PMT are made into the annuity which earns interest per period i over n periods. Since the payments are made into the annuity, the second term is added. The future value of the annuity increases.
If the payments are made from the annuity, the second term is subtracted to give
In this case, the future value of the annuity decreases since money is removed from the annuity. In some applications, we wish to find the present value (what must be in the account today) so that the account ends up with some amount in the future. The next two examples illustrate how to find the present value in cases like this.
Example 1 Find the Amount Needed to Establish a Trust Fund
A wealthy individual wishes to create a trust fund for his grandson so that he may withdraw $5000 at the end of every quarter for ten years. At the end of ten years, the grandson will receive the rest of the trust which contains $50,000. If the trust earns 8% interest compounded quarterly, how much should be put into the trust initially?
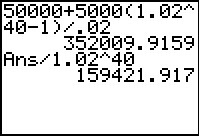
In this problem, the amount in the annuity is decreasing since withdrawals are being made. However, we wish the future value of the annuity to be $50,000 in ten years. This means that a larger amount must be placed in the trust now so that payment may be made from it. Substitute FV = 50,000, PMT = 5000, i = 0.08/4 = 0.02, and n = 4 · 10 = 40 into
to give
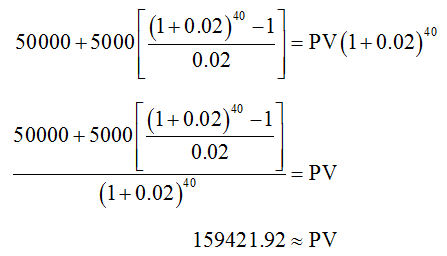
Now solve this equation for the present value.
This is calculated on a TI Graphing Calculator as shown below.
The trust must be established with an initial deposit of $159,421.92.
Example 2 Reaching a Retirement Goal
A fifty-five year old investor wishes to retire at age 67. The investor has budgeted $1000 a month that she may deposit in an ordinary annuity that earns 5% interest compounded monthly. If she wishes to accumulate $2,000,000 for retirement, what must be in the account today to reach that goal?
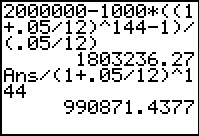
Solution His is an increasing annuity since regular payments are being made an account. Substitute PMT = 1000, i = 0.05/12, n = 12 · 12 = 144 and FV = 2,000,000 into
and solve for the present value PV. This yields
This is calculated on a TI Graphing Calculator as shown below. To simplify the calculation, the numerator is calculated first. Then that answer is divided by the denominator.
There must be $990,871.44 in the annuity today, for the value to grow to $2,000,000 in twelve years.