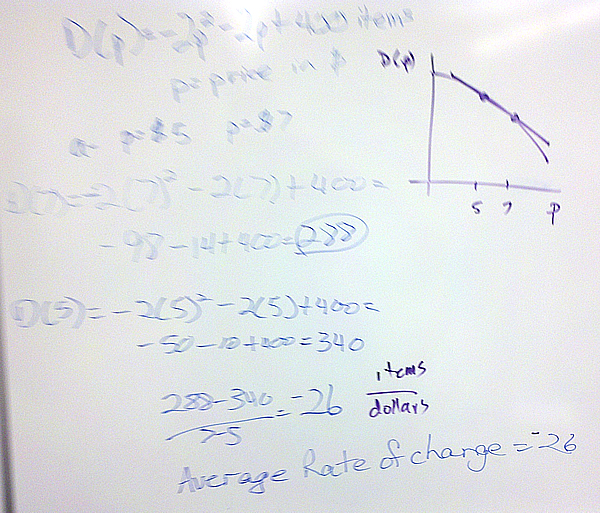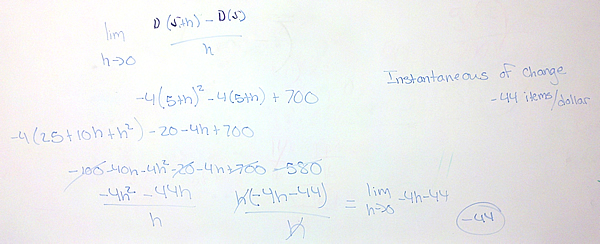Here are several examples where the average rate of change is calculated from some type of economics formula.
Problem 1 The demand for a particular product is given by
$latex \displaystyle D(p)=-2{{p}^{2}}-2p+400\quad \text{items}$
where p is the unit price in dollars.
a. Find the average rate of change of demand with respect to price between a price of 5 dollars and 7 dollars.
b. Find the instantaneous rate of change of demand at a price of 5 dollars.
(Sorry for the shaky cam…too much caffeine!)
The average rate tells us that for each increase in price of 1 dollars between 5 dollars and 7 dollars, the demand for the product drops by 26 items. The instantaneous rate of change tells us that at a price of 5 dollars, the demand is dropping by 22 items per dollar.
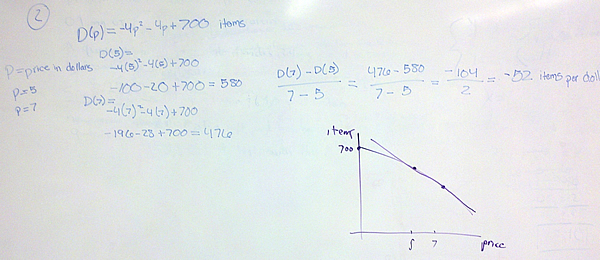
Problem 2 The demand for a particular product is given by
$latex \displaystyle D(p)=-4{{p}^{2}}-4p+700\quad \text{items}$
where p is the unit price in dollars.
a. Find the average rate of change of demand with respect to price between a price of 5 dollars and 7 dollars.
b. Find the instantaneous rate of change of demand at a price of 5 dollars.
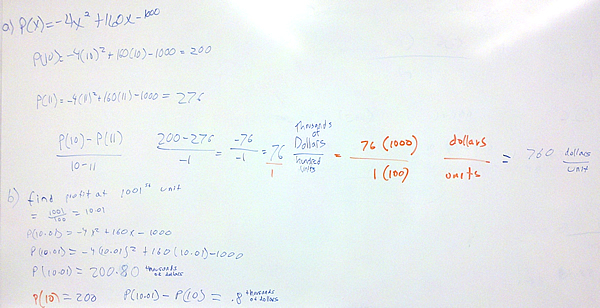
Problem 3 The profit (in thousands of dollars) for selling x hundred units of compressors is
$latex \displaystyle P(x)=-4{{x}^{2}}+160x-1000$
a. Find the average rate of change of profit with respect to compressors from x = 10 to x = 11.
b. Find the exact profit from the 1001st compressor.
c. Find the instantaneous rate of change of profit with respect to compressors at x = 10.
Problem 4 The profit (in thousands of dollars) for selling x hundred units of graphics displays is
$latex \displaystyle P(x)=-5{{x}^{2}}+80x-100$
a. Find the average rate of change of profit with respect to displays from x = 10 to x = 11.
b. Find the exact profit from the 1001st display.
c. Find the instantaneous rate of change of profit with respect to displays at x = 10.
In these last two problems, pay careful attention to the units on the rates. They are all in thousands of dollars per hundred units. This simplifies to tens of dollars per unit since one thousand divided by one hundred is ten.
Also note that to find the profit from the 1001st item, we need to find the profit at a production level of 1001 and subtract the profit at a production level of 1000. This quantity is called the marginal profit at a production level of 1000. As noted in the text, it is approximately equal to the instantaneous rate of change at a production level of 1000.