A tangent line to a function is a line that looks most like the function at a point. In common terms, it just grazes the function.
To find its equation, we need to locate the point where the two meet as well as the slope of the function at that point. Then we can use the slope-intercept form or point-slope form of a line to get the equation.
Since this problem is asking for the equation of a line, let’s start with the point-slope form
This requires a point (x1, y1) and slope m. We’ll use the function to get the point and the derivative to get the slope of the tangent line.
Find the point: We are given a point x = 3. To find the corresponding y value, put the x value into the function
Find the slope of the tangent line: We need h′(3) to get the slope of the tangent line. We’ll use the Power Rule to take the derivative,
The slope of the tangent is
Write the equation of the tangent line: Putting the point (3, 10) and the slope 9 into the line yields
If you are asked to write this in slope-intercept form, you’ll need to solve this for y to give
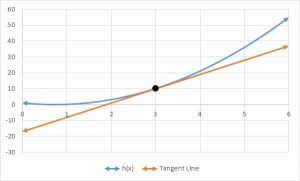
If you graph h(x) and the tangent line together, it should be obvious that your tangent line is correct (ie. tangent).