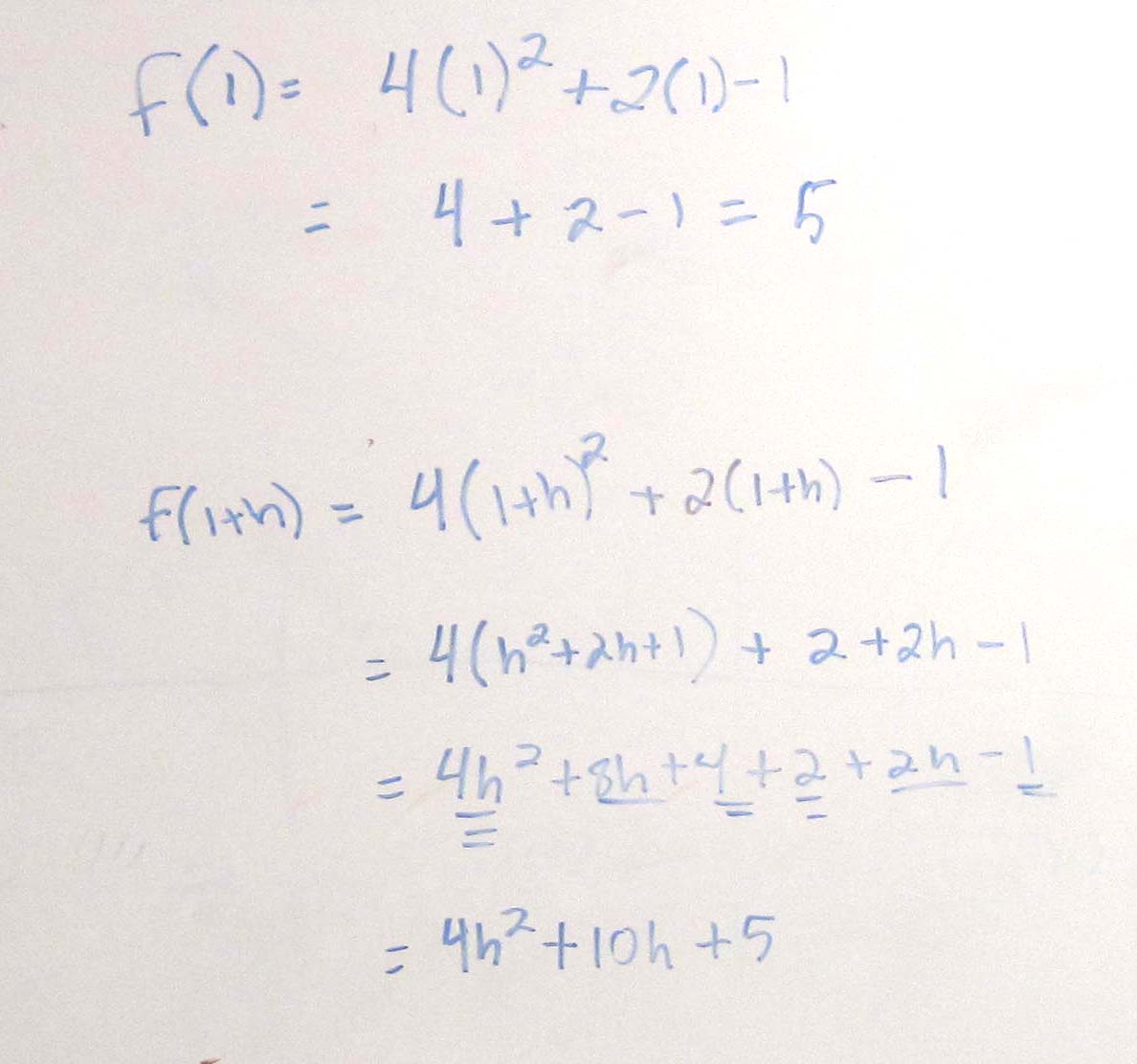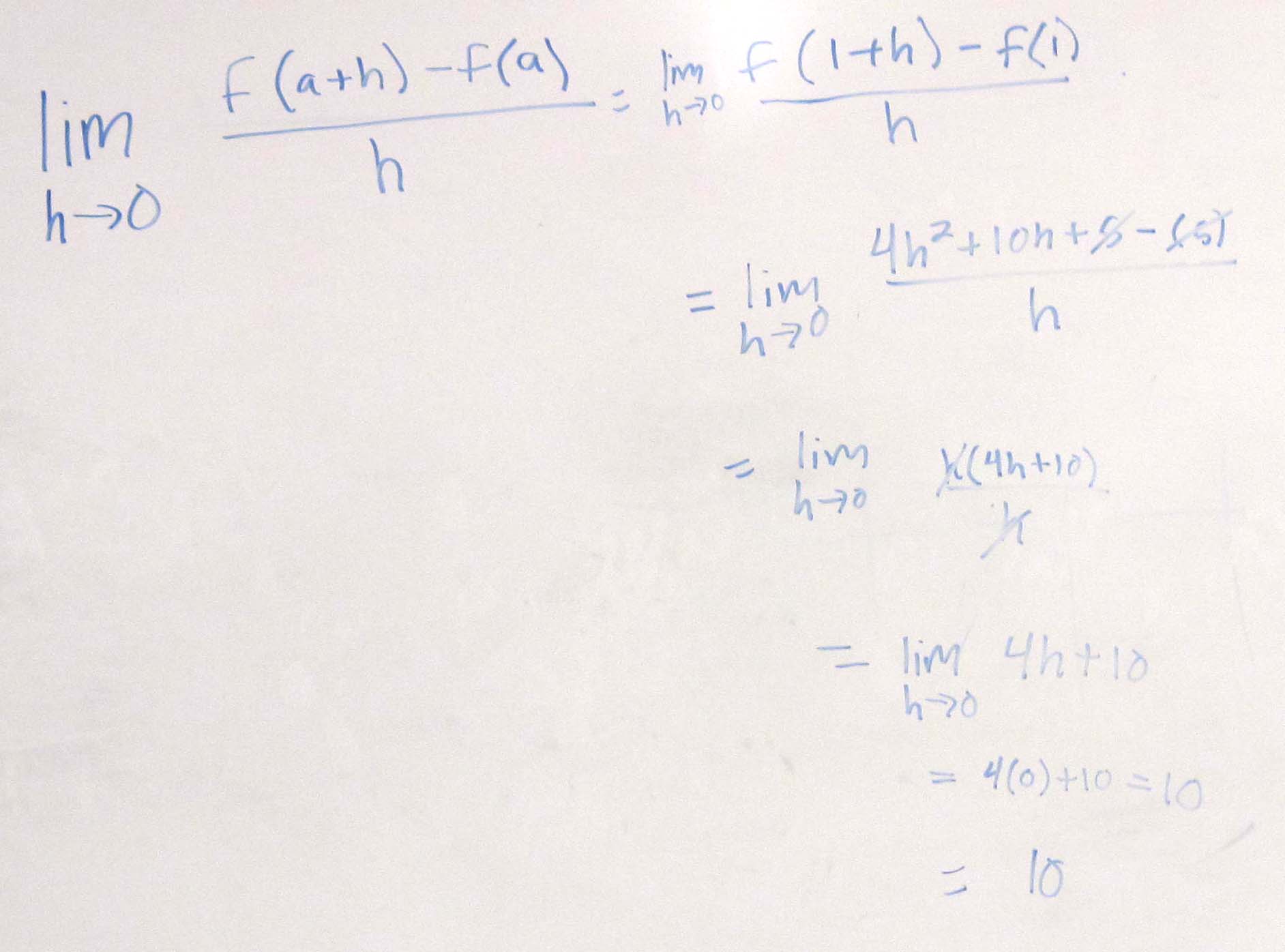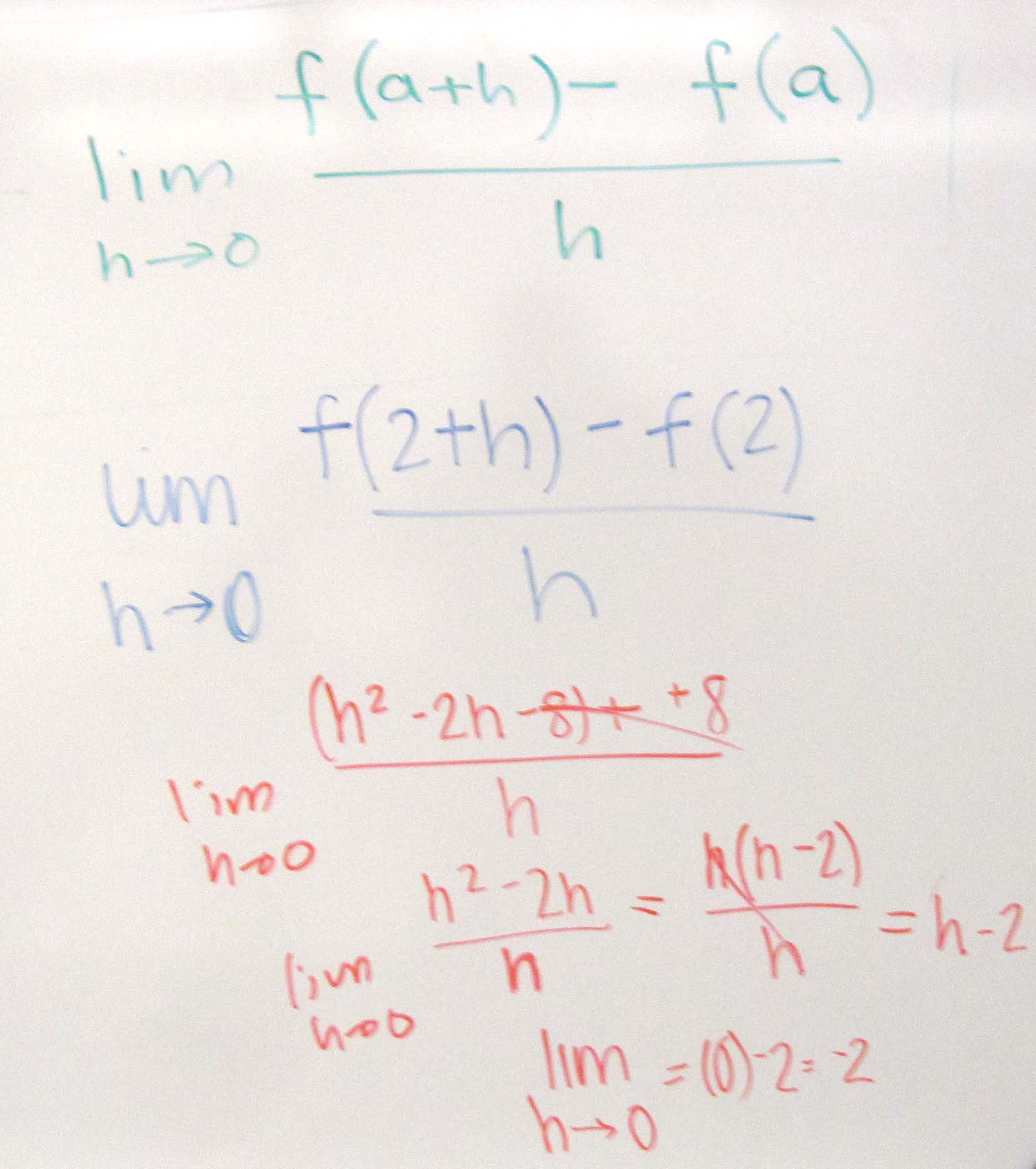The instantaneous rate of change is calculated to find how fast one quantity changes with respect to another.
The instantaneous rate of change of f (x)with respect to x at x = a is
$latex \displaystyle \begin{matrix}
\text{Instantaneous rate of change of }f\text{ } \\
\text{with respect to }x\text{ at }x=a \\
\end{matrix}=\underset{h\,\,\to 0}{\mathop{\lim }}\,\frac{f(a+h)-f(a)}{h}$
To apply this definition, you need to identify the point a at which the rate is to be calculated. Then the function values f (a) and f (a+h) are calculated and simplified. Finally, these are substituted into the limit so that it evaluated.
Example 1 Find the instantaneous rate of change of $latex \displaystyle f(x)=4{{x}^{2}}+2x-1$ at $latex \displaystyle x=1$.
Solution Start by calculating the two function values.
Once you have the function values, substitute them into the definition for instantaneous rate of change.
Example 2 Find the instantaneous rate of change of $latex \displaystyle f(x)={x}^{2}+6x$ at $latex \displaystyle x=2$.
Solution The function values are
Now put these into the limit definition of instantaneous rate of change.
