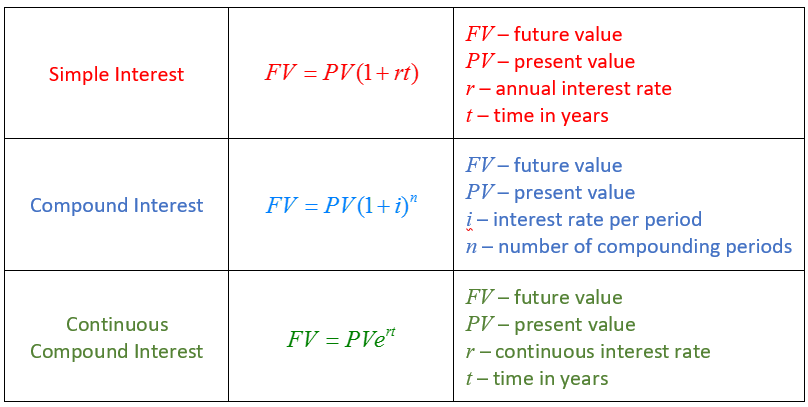
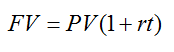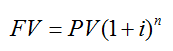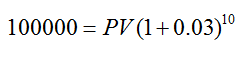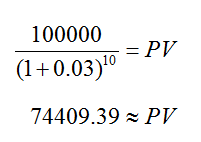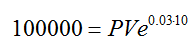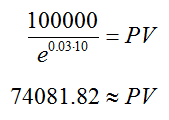In many investment problems, you are given an amount of money and asked what will it accumulate to in a certain amount of time at some interest rate. Essentially, these problems are asking you to find the future value of the amount of money. Depending on how that money accumulates, you might use one of several different formulas.
If the problem specifies simple interest, you would use
where an amount PV accumulates to a future value FV in t years at a rate of r.
If the problem specifies compound interest, you would use
where i is the interest rate per period and n is the number of periods.
It is also possible for a problem to specify continuous compounding in which case you would use
where r is the continuous rate and t is the number of years.
For a future value problem, the quantities on the right side of each of these equations will be specified so that you can calculate the future value FV.
In a present value problem, you will be given the amount in the future FV and asked to find the amount you would start with to get to that amount. Let’s look at two possible problems.
Problem What would need to be deposited today to reach a value 0f 100,000 dollars in 10 years at a rate of 3% per year compounded annually?
Solution The first thing we need to realize is which formula we need to use. Since it says the interest is compounded annually, we need
In the compound interest formula we are given that the future value is 100,000 dollars, the interest rate per period is i = 0.03, and the money will accumulate over 10 periods. Putting these values into the compound interest formula yields
Solving this equation for PV yields
This means that the present value of 100,000 dollars is approximately 74,409.39 dollars when compounded annually for 10 years at an annual rate of 3%.
Now let’s change the problem up and see how this affects the solution process.
Problem What is the present value of 100,000 dollars compounded continuously at a rate of 3% per year?
Solution On the surface, this is almost the same problem as the one above except that it specifies continuous interest. This means we need to start from
instead of the compound interest formula. Putting in the values in the problem yields
Isolating the present value PV gives
In each of these problems, we need to solve for the present value PV but we start from a different formula that depends on the how interest is earned.