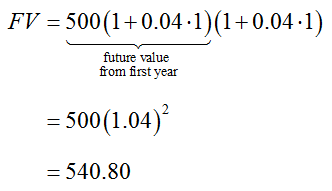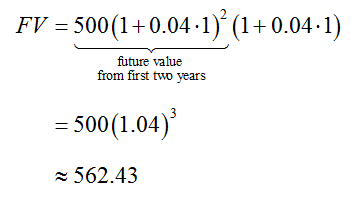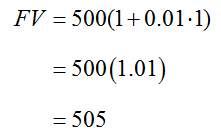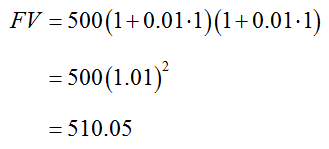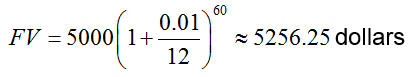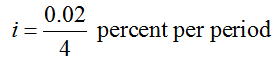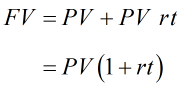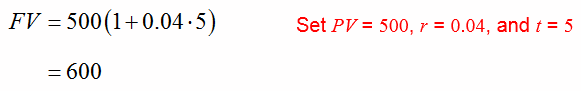Category: Finite Mathematics Ebook

What is compound interest?

In a loan or investment earning compound interest, interest is periodically added to the present value. This additional amount earns interest. In other words, the interest earns interest.

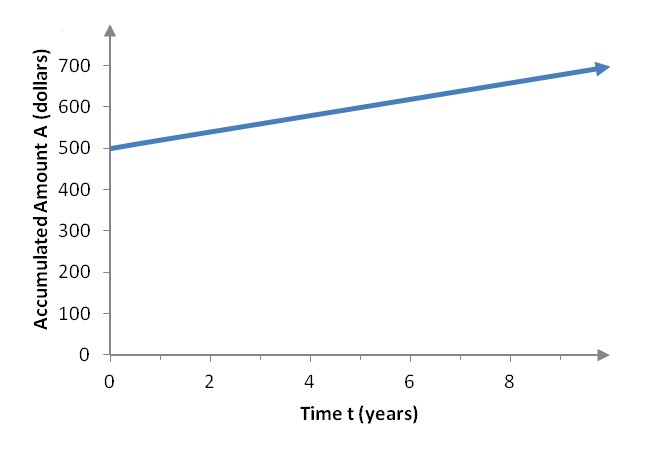
Let us illustrate this process with a concrete example. Suppose we deposit $500 in an account that earns interest at a rate of 4% compounded annually. This rate is the nominal or stated rate. By saying that interest is compounding annually, we mean that interest is added to the principal at the end of each year.

For instance, we use the simple interest formula, FV = PV (1 + rt), to compute the future value at the end of the first year,

To find the future value at the end of the second year, we let the present value be the future value from the end of the first year in the simple interest formula,

Since the present value in this amount includes the interest from the first year, the interest from the first year is earning interest. This is the effect of compounding.

To find the future value at the end of the third year, we let the future value at the end of the second year be the present value in the simple interest formula,

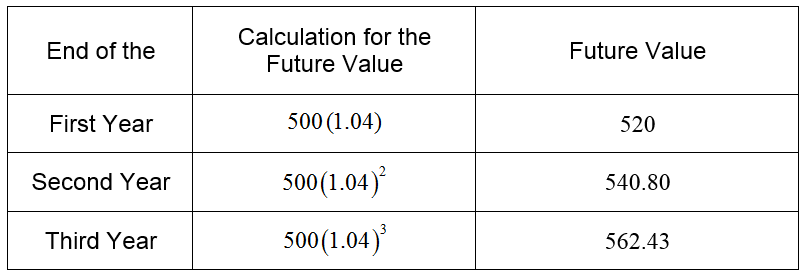
Let us summarize these amounts in a table.

The middle column establishes a simple pattern. At the end of each year, the future value is equal to the present value times several factors of 1.04. These factors correspond to the compounding of interest.

In general, if interest is compounded annually, then the future value is

where PV is the principal, r is the nominal rate and t is the time in years.

If interests compounds more than once a year, finding the future value is more challenging. It is more likely that interest is compounded quarterly (4 times a year), monthly (12 times a year) or daily (365 times a year). The length of time between which interest is earned is the conversion period. The length of time over which the loan or investment earns interest is the term. To account for compounding over shorter conversion periods, we need more factors in the expression for the future value. However, in each of these factors we only earn a fraction of the interest rate.

For instance, suppose deposit $500 in an account earning 4% compounded quarterly. To calculate the future value, we multiply the principal by a factor corresponding to one-fourth of the interest rate each quarter. The future value after one quarter is

After two quarters, the future value contains two factors corresponding to one percent interest per quarter,

Continue this pattern for twelve conversion periods (twelve quarters or three years) gives

If we compare this expression to the expression for compounding quarterly, FV = 500(1.04)³, we note several differences. When we compound quarterly, we get four times as many factors in the future value. This is due to the fact compounding quarterly means we need four times as many factors. When we compound quarterly, each factor utilizes a rate that is one-fourth the rate for compounding annually.

You may also see compound interest computed from the formula  This is the exact same formula as the one above except the present value is called the principal P and the future value is called the accumulated amount A.

Example 1         Compound Interest

A customer deposits $5000 in an account that earns 1% annual interest compounded monthly. If the customer makes no further deposits or withdrawals from the account, how much will be in the account in five years?

Solution To utilize the compound interest formula, FV = PV (1 + i)ⁿ, we must find the present value PV, the interest rate per conversion period i, and the number of conversion periods n. The present value or principal is the amount of the original deposit so . The account earns 1% annual interest, compounded monthly. This means the account earns

over each conversion period. Since the interest is compounded monthly over 5 years, there are n = 12 · 5 or 60 conversion periods during the time this money is deposited. The future value is

---

If the future value, interest rate, and number of conversion periods is known, we can solve for the present value in FV = PV (1 + i)ⁿ. In problems like this, we want to know what amount should we start with to grow to a known future value.

Example 4      Present Value

A couple needs $25,000 for a large purchase in five years. How much must be deposited now in an account earning 2% annual interest compounded quarterly to accumulate this amount? Assume no further deposits or withdrawals during this time period.

Solution To find the amount needed today, we must find the present value of $25,000. The interest for each conversion period is

The account earns interest over a total of 4·5 or 20 conversion periods. Substitute these values into the compound interest formula, FV = PV (1 + i)ⁿ, and solve for PV:

We round the present value in the last step to two decimal places. This ensures the value is accurate to the nearest cent. If the couple invests $22,626.57 for five years, it will grow to $25,000 at this interest rate.

What is simple interest?

In business, individuals or companies often borrow money or assets. The lender charges a fee for the use of the assets. Interest is the fee the lender charges for the use of the money. The amount borrowed is the principal or present value of the loan.

Simple interest is interest computed on the original principal only. If the present value PV, in dollars, earns interest at a rate of r for t years, then the interest is

I = PV rt

The future value (also called the accumulated amount or maturity value) is the sum of the principal and the interest. This is the amount the present value grows to after the present value and interest are added.

The interest rate r is the decimal form of the interest rate written as a percentage. This means an interest rate of 4% per year is equivalent to r = 0.04.

In this text, we use the variable names commonly used in finance textbooks. Instead of writing the present value as the single letter P, we use two letters, PV. Be very careful to interpret this as a single variable and not a product of P and V. Similarly, the future value is written FV. This set of letters represents a single quantity, not a product of F and V. This allows us to use groups of letters to represent quantities that suggest their meaning.

If we know two of the quantities in this formula, we can solve for the other quantity. This formula is also used to calculate simple interest paid on investments or deposits at a bank. In these cases, we think of the deposits or investment as a loan to the bank with the interest paid to the depositor.

Example 1         Simple Interest

An investment pays simple interest of 4% per year. An investor deposits $500 in this investment and makes no withdrawals for 5 years.

a.  How much interest does the investment earn over the five-year period?

Solution Use I = PV rt to compute the interest

b.   What is the future value of the investment in 5 years?

Solution The future value is computed using FV = PV (1 + rt),

c.   Find an expression for the future value if the deposit accumulates interest for t years. Assume no withdrawals over the period.

Solution In this part, the time t is variable,

This relationship corresponds to a linear function of t. The vertical intercept is 500 and the slope is 20. This tells us that the initial investment is $500 and the accumulated amount increases by $20 per year.

Figure 1 – The linear function describing the accumulated amount in Example 1c.

---

Example 3      Simple Interest

A small payday loan company offers a simple interest loan to a customer. They will loan the customer $750. The customer promises to repay the company $808 in two weeks. What is the annual interest rate for this loan?

Solution Since there are 52 weeks in a year, the length of this loan is ²⁄₅₂ years. Use the information in the problem in the simple interest formula, FV = PV (1 + rt), to solve for the rate r:

This decimal corresponds to an interest rate of 201% per year. Because of such high rates, many states are passing legislation to limit the interest rates that pay day loan companies charge.

Section 1 – Discrete Probability Distributions

Section 2 – Binomial Probability Distribution

Section 3 – Poisson Probability Distribution

Section 4 – Continuous Probability Distribution

Section 5 – Uniform and Exponential Probability Distribution

Probability with Permutations and Combinations

In sections 8.1 and 8.2, you learned about counting objects using the Multiplication Principle, permutations, and combinations. With these strategies, we are able to count the number of different license plate numbers or ways to select lottery numbers. In this section you’ll go one step further and use these strategies to find probabilities.

The key to finding these probabilities is an assumption. We will assume that the outcomes in whatever event and experiment we are considering are equally likely. This will enable us to calculate the probability of an event by counting the number of outcomes in it. Specifically, we’ll find the probability of an event E from a sample space S with

where n(E) and n(S) are the number of outcomes in E and S. This will helps us to calculate the likelihood (or unlikelihood) of events such as winning the lottery jackpot or detecting a defective product on a production line.

Read in Section 8.3

• How do you find the likelihood of a certain type of license plate?
• How do you find the likelihood of a particular committee?
• How do you find the probability of winning a lottery?
• How do you find the likelihood of detecting a defective product?

Section 8.3 Workbook (PDF) – 9/4/19

Watch Video

Combinations

In Section 8.1, we used permutations to count different arrangements of objects. The word “arrangements” is used since different orders of objects must be counted separately. Different arrangements of numbers and letters on an auto license plate lead to different license plate numbers.

In many applications, different arrangements of objects are not counted differently. Many states have lotteries that are used to fund schools and environmental causes. In these lotteries, lottery officials select numbered balls from a group of balls labeled 1 through a larger number like 52. A player wins the lottery jackpot if the numbers the player selects matches the numbers on the balls selected by officials. The order in which the balls are drawn does not have to be duplicated. The number of ways the balls can be selected from a larger group of balls is calculated using combinations. In combinations, groupings of objects are counted and the order of the objects in the grouping are irrelevant.

---

Read in Section 8.2

• What is a combination?
• What is the difference between a permutation and combination?

Section 8.2 Workbook (PDF) – 9/4/19

Watch Videos